[Func-Num] 7.7 Quadratic Equations and Applications

📋 This is my note-taking from what I learned in the class “Math175-002 Functions & Number Systems”

Objectives

1. Recognize a quadratic equation in standard form.
2. Use the zero-factor property to solve a quadratic equation (when applicable).
3. Use the square root property to solve a quadratic equation (when applicable).
4. Use the quadratic formula to solve a quadratic equation.
5. Solve an application that leads to quadratic equations.

Quadratic Equations and Applications

1. Quadratic Equations
2. Zero-Factor Property
3. Square Root Property
4. Quadratic Formula
5. Applications

1. Quadratic Equations

We have considered linear equations and inequalities in earlier sections. Now we investigate second-degree, or quadratic, equations.

An equation that can be written in the form

Standard form: ax² + bx + c = 0

where a, b, and c are real numbers, with a≠0, is a quadratic equation. The form of the equation given above is called standard form.

2. Zero-Factor Property

One method of solving a quadratic equation depends on the following property.

If ab = 0, then a = 0 or b = 0 or both.

Solving a quadratic equation by the zero-factor property requires that the equation be in standard form before factoring.

Example: Zero-Factor Property

Solve 6x² + 7x = 3.

Solution
6x2 + 7x = 3	Given equation
6x2 + 7x - 3 = 0	Write in standard form
(3x - 1)(2x + 3) = 0	Factor
3x - 1 = 0 or 2x + 3 = 0	Zero-factor property
3x = 1 or 2x = -3	Solve each equation
x = 1/3 or x = -3/2	Divide

Check. Substitute \({1} \over {3}\) and - \({3} \over {2}\) in the original equation. The solution set is { \({1} \over {3}\) , \({3} \over {2}\) }

3. Square Root Property

If k >= 0, then the solutions of x² = k are + $\sqrt {k}$ or - $\sqrt {k}$

• If k > 0, the equation x² = k has two real solutions.
• If k = 0, there is only one solution, 0.
• If k < 0, there are no real solutions → (In this last case, there are complex solutions. Complex numbers are covered in more extensive algebra texts.)

A quadratic equation of the form x² = k, k >= 0, can be solved as follows.

Solution
x2 = k	Given equation
x2 - k = 0	Subtract k
(x + sqrt{k})(x - sqrt{k}) = 0	Factor, using radicals
x + sqrt{k} = 0 or x - sqrt{k} = 0	Zero-factor property
x = - sqrt{k} or x = sqrt{k}	Solve each equation

This leads to the square root property for solving equations.

Example: Square Root Property

Solve each quadratic equation for real solutions.

a) x² = 36 → The solution set is {+6, -6}

b) (x - 1)² = 8 → The solution set is {1 + 2(sqrt{2}), 1 - 2(sqrt{2})}

c) m² = -5 → No real solutions, the solution set is ∅.

4. Quadratic Formula

By completing the square (see the margin note at left), we can derive one of the most important formulas in algebra, the quadratic formula.

The solution of $(ax^2 + bx + c = 0)$, $a \ne 0$, are given by the quadratic formula.

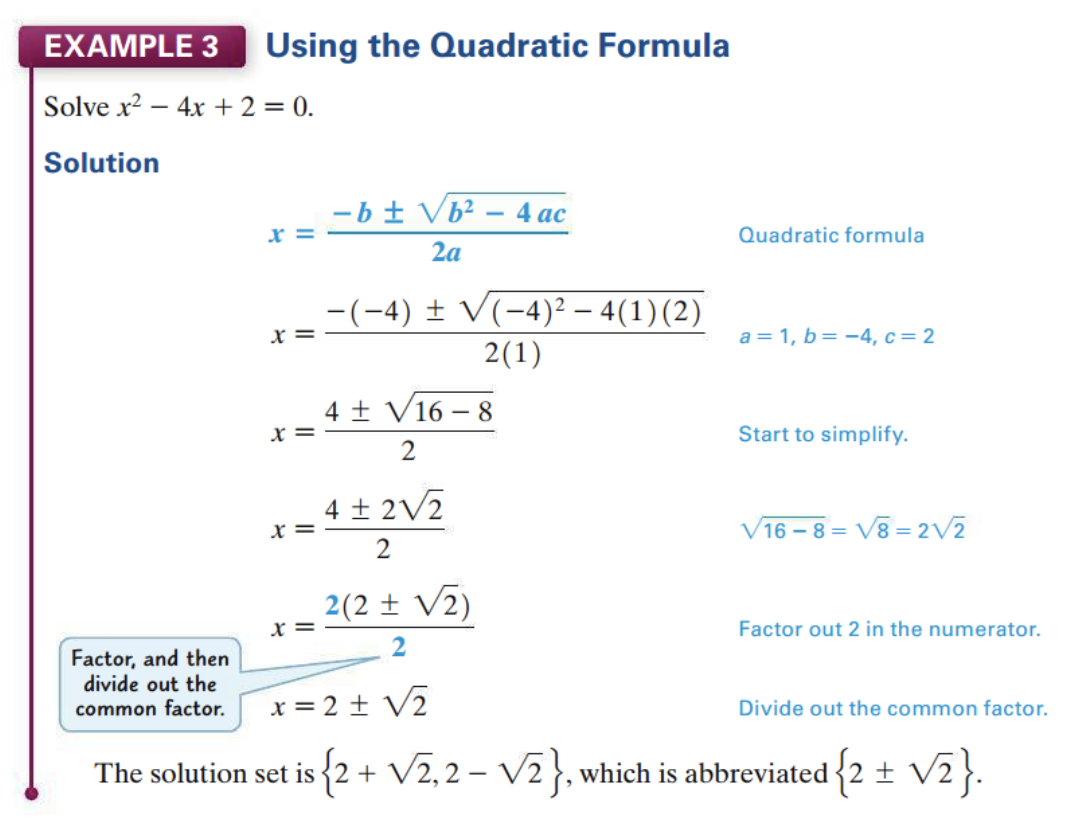
\[x = {-b \pm \sqrt{b^2-4ac} \over 2a}\]

Example: Quadratic Formula

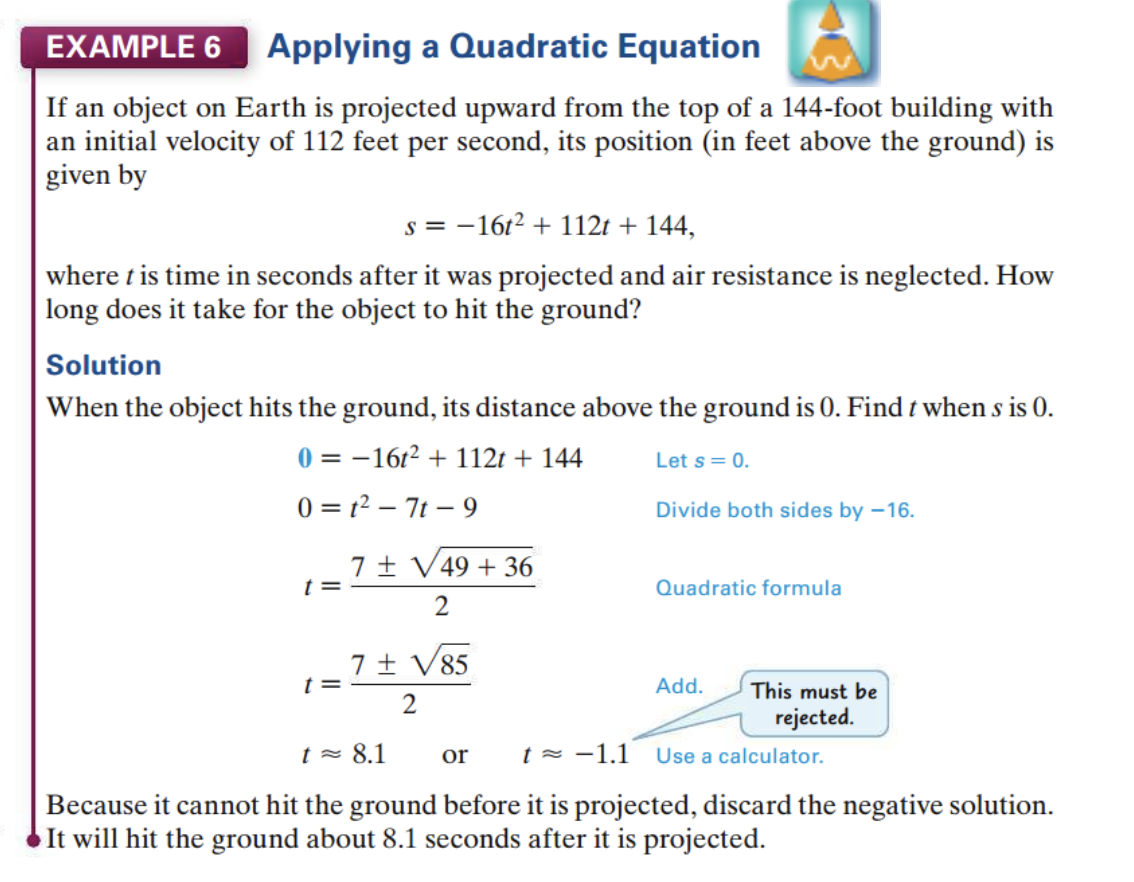
5. Applications

Exercise

Section 7.7: 1~39 (odds)

---

Back to Top

See other articles in Category Func-Num