[Func-Num] 7.1 Special Kinds of Linear Equations

📋 This is my note-taking from what I learned in the class “Math175-002 Functions & Number Systems”

Special Kinds of Linear Equations

Type	Number of Solutions	Final Line When Solving
Conditional	One	Final line is x = a number
Identity	Infinite; solution set {all real numbers}	Final line is True statement, such as 0 = 0.
Contradiction	None; solution set ∅	Final line is False statement, such as 0 = 1.

Example: Conditional

Solve 5x - 9 = 4(x - 3).

Solution
5x - 9 = 4(x - 3)	Original equation
5x - 9 = 4x - 12	Distributive property
5x - 9 - 4x = 4x - 12 - 4x	Subtract 4x
x - 9 = -12	Combine like terms
x - 9 + 9 = -12 + 9	Add 9
x = -3	Solution set {-3}

The solution set has one element, so 5x - 9 = 4(x - 3) is a “conditional equation”.

Example: Identity

Solve 5x - 15 = 5(x - 3).

Solution
5x - 15 = 5(x - 3)	Original equation
5x - 15 = 5x - 15	Distributive property
0 = 0	Subtract 5x and add 15

The final line, 0=0, indicates that the solution set is {all real numbers}, and the equation 5x - 15 = 5(x - 3) is an “identity”. (Note: The first step yielded 5x - 15 = 5x - 15, which is true for all values of x, implying an identity there.)

Example: Contradiction

Solve 5x - 15 = 5(x - 4).

Solution
5x - 15 = 5(x - 4)	Original equation
5x - 15 = 5x - 20	Distributive property
5x - 15 - 5x = 5x - 20 - 5x	Subtract 5x
-15 = -20	False

Because the result, -15 = -20, is false, the equation has no solution. The solution set is ∅, and the equation is a “contradiction”.

Literal Equations and Formulas

An equation involving variables (or letters), such as cx+d=e, is called a “literal equation”. The most useful examples of literal equations are formulas. The solution of a problem in algebra often depends on the use of a mathematical statement or “formula” in which more than one letter is used to express a relationship.

Examples of formulas

In some cases, a formula must be solved for one of its variables. This process is called “solving for a specified variable”. The steps used are similar to those used in solving linear equations.

Solving for a Specified Variable

When you are solving for a specified variable, the key is to treat that variable as if it were the only one. Treat all other variables like numbers (constants).

• Step 1

  If the equation contains fractions, multiply both sides by the LCD to clear the fractions.

• Step 2

  Transform so that all terms with the specified variable are on one side and all terms without that variable are on the other side.

  If necessary, use the distributive property to combine the terms with the specified variable.

• Step 3

  Divide each side by the factor that is the coefficient of the specified variable. (Divide both sides by the factor that is multiplied by the specified variable.)

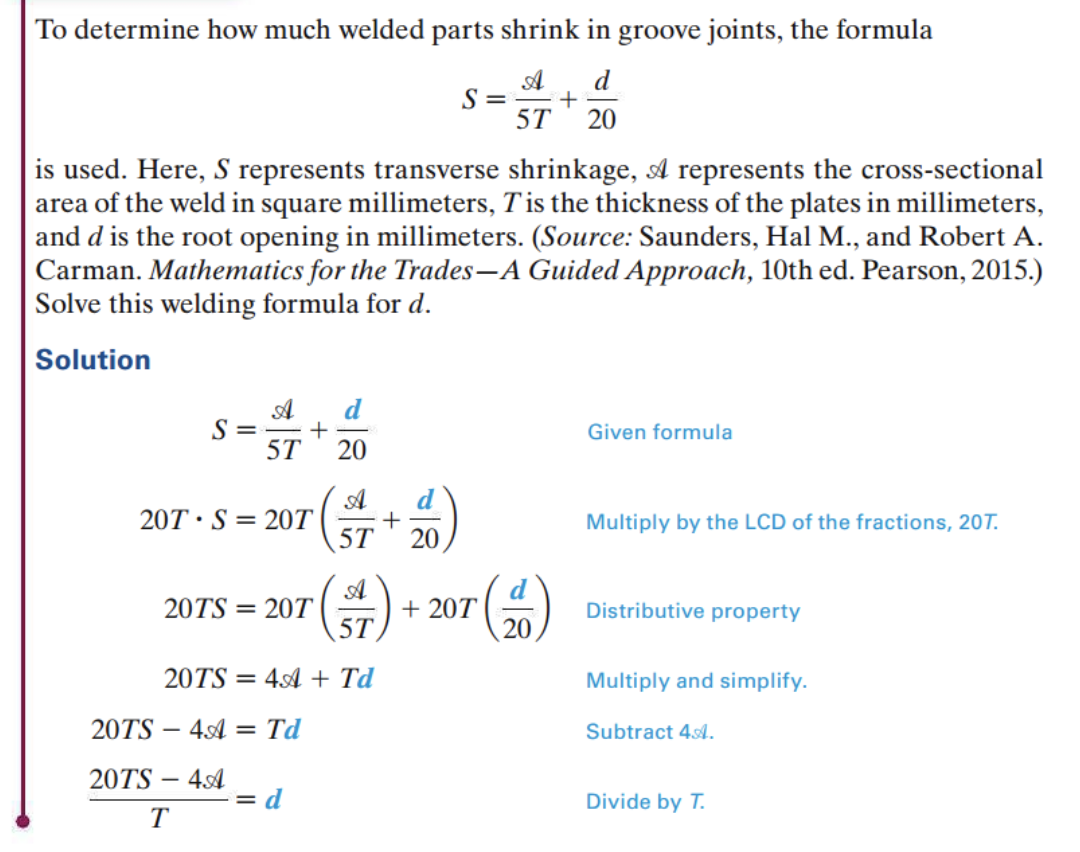
Example: Solving for a Specified Variable

Solve the formula “P = 2L + 2W” for L.

Solution
P – 2W = 2L + 2W – 2W	Subtract 2W
P – 2W = 2L	Combine like terms
\({P – 2W} \over {2}\) = \({2L} \over {2}\)	Divide by 2

The result is

• \({P – 2W} \over {2}\) = L
• \({P} \over {2}\) - W = L

Models

An equation or an inequality that expresses a relationship among various quantities is an example of a “mathematical model”. The relationship between the Fahrenheit and Celsius temperature scales is an example of a linear model.

A mathematical model is an equation (or inequality) that describes the relationship between two quantities. A linear model is a linear equation.

Example: Using the Formulas for Fahrenheit and Celsius

The relationship between degrees Celsius (C) and degrees Fahrenheit (F) is “modeled by the linear equation” “F = 1.8C + 32.”

What Celsius degree corresponds to a Fahrenheit reading of 50°F?

Solution
Because F = 50, the equation becomes	50 = 1.8C + 32
500 = 18C + 320	Multiply by 10
180 = 18C	Subtract 320
C = 10	Divide by 18

Therefore, a reading of “50 degrees Fahrenheit” corresponds to a reading of “10 degrees Celsius”.

Exercise

Section. 7-1: 1 – 31, 51 – 61 (odds)

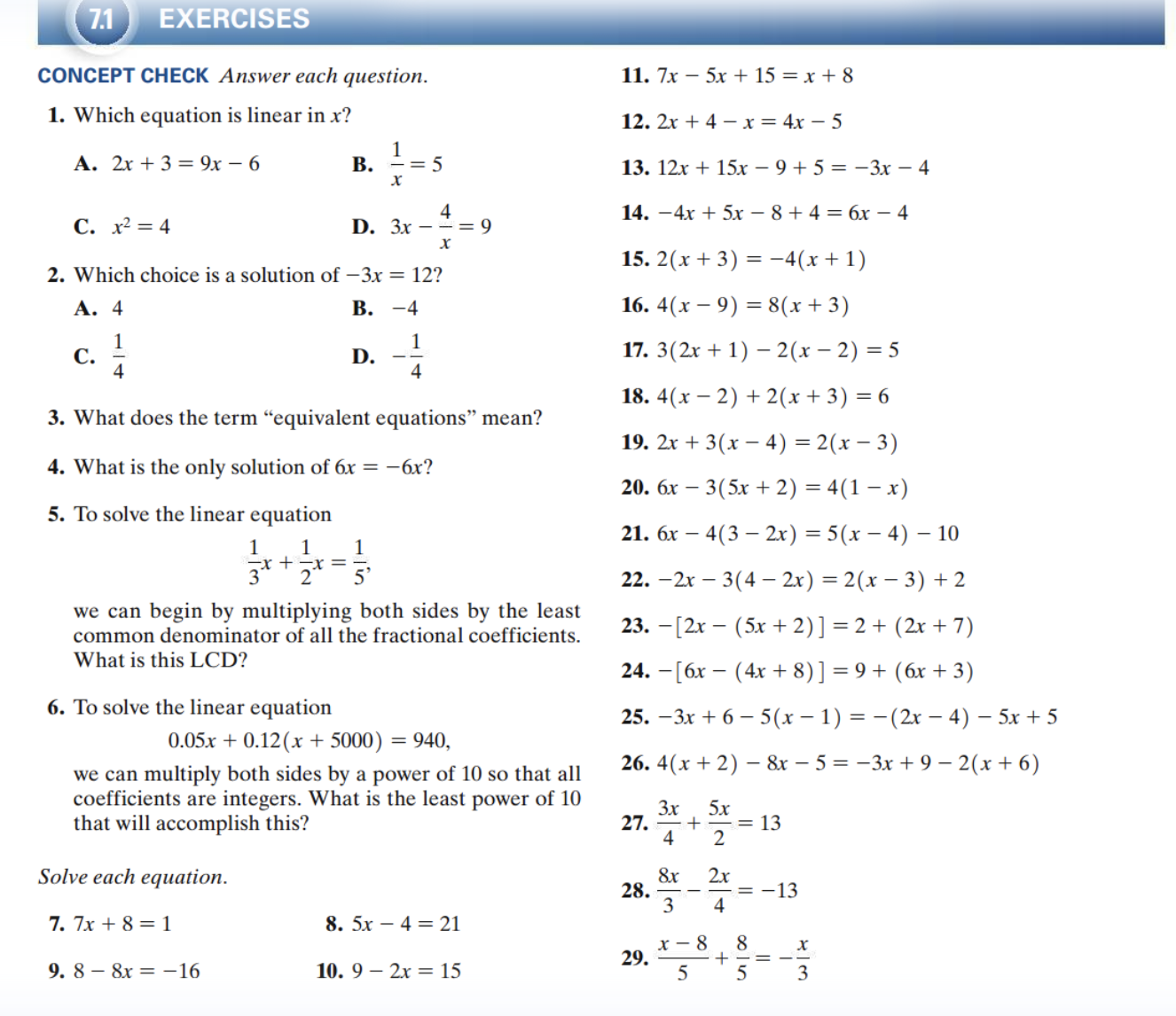
Answer each question.

• 1: Which equation is linear in x ?

  • A. 2x + 3 = 9x -6 → ○

  • B. 1/x = 5 → ✕

  • C. x² = 4 → ✕

  • D. 3x - 4/x = 9 → ✕

• 3: What does the term “equivalent equations” mean? → They have the same solution set.
• 5: To solve the linear equation \({1}x \over {3}\) + \({1}x \over {2}\) = \({1} \over {5}\) we can begin by multiplying both sides by the least common denominator of all the fractional coefficients. → What is this LCD? → 30

Solve each equation.

• 7: Solution set x is -1
• 9: Solution set x is 3
• 11: Solution set x is -7
• 13: Solution set is empty set, and the equation is “Contradiction”
• 15: Solution set x is -5/3
• 17: Solution set x is -1/2
• 19: Solution set x is 2
• 21: Solution set x is -2
• 23: Solution set x is 7
• 25: Solution set x is 2
• 27: Solution set x is 4
• 29: Solution set is empty set, and the equation is “Contradiction”

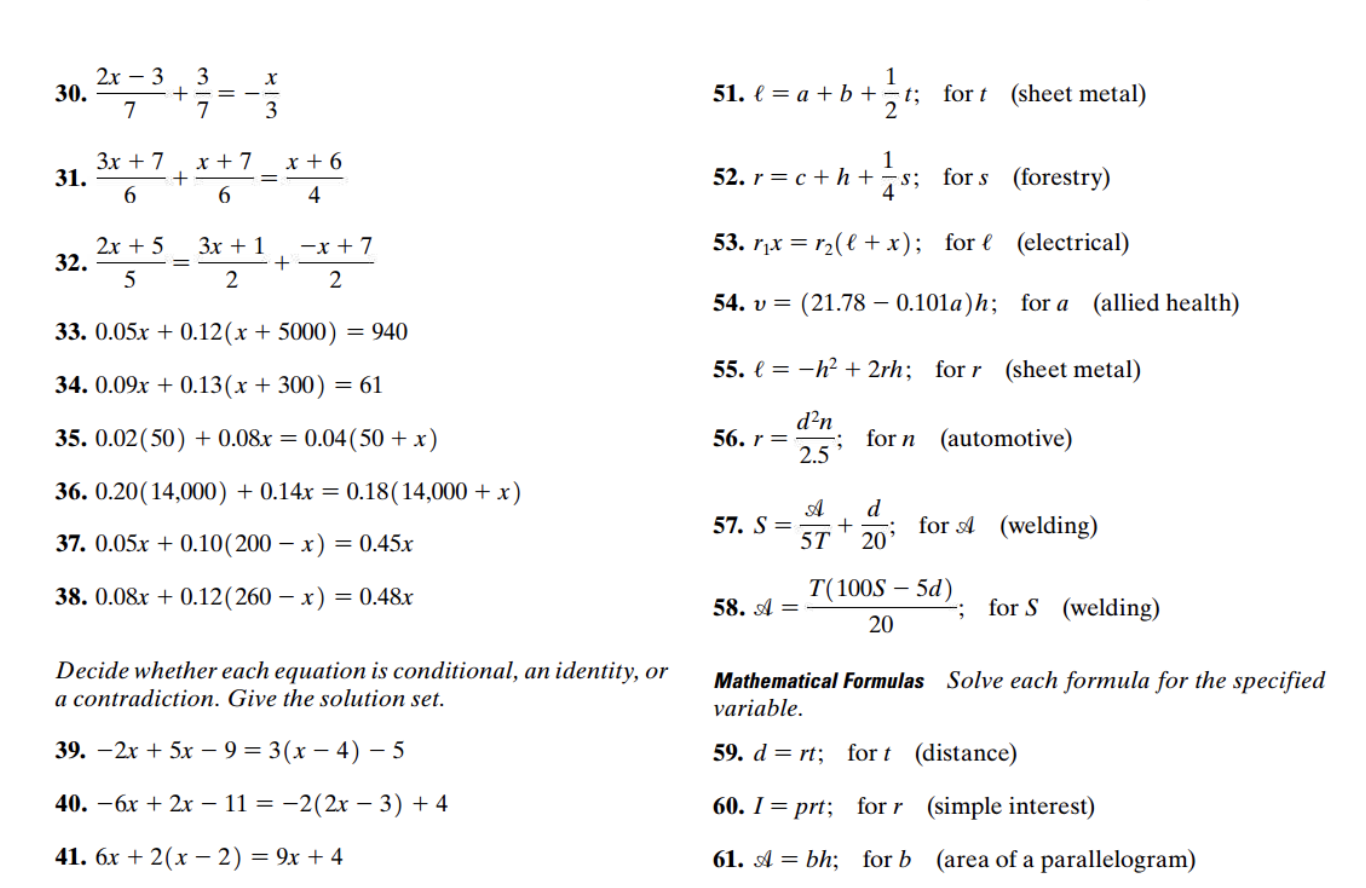
• 31: Solution set x is -2

Formulas from Trades and Occupations: The formulas here are found in various trades and occupations. Solve each formula for the specified variable. (Sources: Saunders, Hal M., and Robert A. Carman. Mathematics for the Trades—A Guided Approach, 10th ed. Pearson, 2015; Timmons, Daniel L., and Catherine W. Johnson. Math Skills for Allied Health Careers. Pearson, 2008.)

• 51: t = 2(l - a - b)
• 53: l = \({r_1}x \over {r_2}\) - x
• 55: r = \({l + h^2} \over {2h}\)
• 57: A = 5T(S - \({d} \over {20}\) )

Mathematical Formulas: Solve each formula for the specified variable.

• 59: t = \({d} \over {r}\)
• 61: b = \({A} \over {h}\)

---

Back to Top

See other articles in Category Func-Num